ANALYSIS OF THE SCALE EFFECTS ON COMBINATIONS OF PREPOSITIONS ON POSITIONING OF THE LO
The different sizes of the boundaries that we cosidered here were:
Case 1: 700x700 sq. units
Case 2: 600x600 sq. units
Case 3: 500x500 sq.units
Case 4: 400x400 sq. units
In the tests run, the RO was a squre of fixed size.
The size of the LO was taken to be the same.
We performed the test for three combinations of prepositions
Thus in a particular suite of tests corresponding to a
combination of prepositions, the only independent variable was the size
of the boundary.
The dependent variable was the position of the sweet spot.
To see the conclusion, press here.

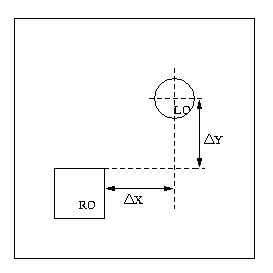
Figure 1 : Test Screen
On performing ANOVA for the ratio dY/dX , we obtained the following results :
|
MEAN |
MEAN |
MEAN |
MEAN |
F-RATIO |
|
0.77 |
0.98 |
0.95 |
1.16 |
0.357 |
The mean of these values is 0.97. The method we have been following so far for combinations of prepositions has been to superpose the fields for the individual prepositions. By this method, given this combination of prepositions the expected value of dY/dX is 1.0. The value obtained from our experiment is very close to the expected value.
Note : For the first case the mean comes out to be 0.77 which is rather low. This is most likely because of the fact that some subjects seem to have misread the instructions given: placing the LO to the right instead of placing it to the front and the right which gives negative values. Removing such values from the analysis would give more coherent results.
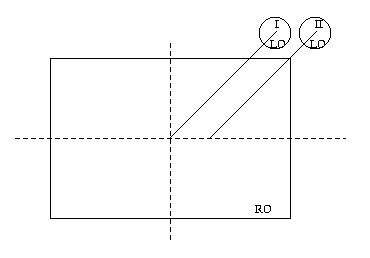
Figure 2
There still remains one question which needs to be studied. In case of combinations of prepositions like front and right, LO is placed along line I or line II (see Figure 2). This must be further studied in detail.
We start off with the assumption that the Y-Coordinate of the centre of the LO will not vary as the boundary changes and is the same as the Y-Coordinate of the centre of the RO. This can be concluded from our analysis of previous results e.g. shape effects of LO. Even for this suite of tests we have verified this independence but the analysis is not shown here for sake of conciseness.
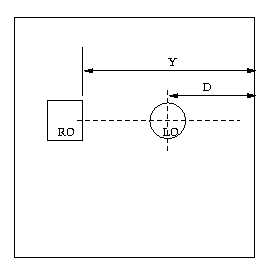
Figure 3 : Test Screen
On performing ANOVA for the distance of the centre of the LO from the boundary , we obtained the following results :
|
MEAN |
MEAN |
MEAN |
MEAN |
F-RATIO |
|
116 |
88 |
70 |
50 |
4.123 |
We tabulate the values of D and Y (as defined in Figure 1) for different sizes of the boundaries
| D | 116 | 88 | 70 | 50 |
| Y | 490 | 400 | 310 | 220 |
We performed linear regression on the above data obtained and calculated D as a function of Y.
D = k + 0.24*Y
where k is a constant
From the above result, we conclude that the location of centre of LO depends on the size of the available space Y.
We start off with the assumption that the Y-Coordinate of the centre of the LO will not vary as the boundary changes and is the same as the Y-Coordinate of the centre of the RO. This can be concluded from our analysis of previous results e.g. shape effects of LO. Even for this suite of tests we have verified this independence but the analysis is not shown here for sake of conciseness.
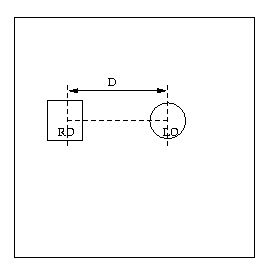
Figure 4 : Test Screen
On performing ANOVA for the distance D of the centre of the LO from the centre of the RO, which is almost the same as the difference of the X-Coordinates of the centres of the RO and the LO since the Y-Coordinate of the centre of the RO remains almost the same as the Y-Coordinate of the centre of the LO, we obtained the following results :
|
MEAN |
MEAN |
MEAN |
MEAN |
F-RATIO |
|
103 |
105 |
102 |
100 |
0.064 |
We observe that D is almost a constant. The very low value of the F-ratio also suggests the validity of this conclusion as the distance between the centres does not vary as the size of the boundary changes.
We hypothesise that this constant is a function of the size of the LO. This must be studied in detail later.
From the above analysis, we conclude that :